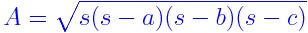Deriving Herons Formula

Category: Plane Geometry, Trigonometry, Algebra

"Published in Newark, California, USA"

Given the triangle below:

Photo by Math Principles in Everyday Life

Prove that  the area of triangle is 

Solution:

Since the given triangle has no altitude, we have to assign it anywhere from the vertices of a given triangle. From point B, draw a line that is perpendicular to b and label this as h as the altitude. 

Photo by Math Principles in Everyday Life

We know that the area of triangle is 

but 

Square the both sides of the equation

but 

From Cosine Law,

Square the above equation

Therefore,

You notice that we can factor the above equation by the difference of two squares

Arrange the above equation and you notice that we can factor again by the difference of two squares

If a + b + c is the perimeter of a triangle, then s is the semi-perimeter of a triangle. Substitute

to the above equation. Therefore,

The above formula is called Heron's Formula. 

Angle Bisector - Two Intersecting Lines

Category: Analytic Geometry, Algebra

"Published in Newark, California, USA"

Find the equation of a line that bisects the acute angle formed by the following lines:

         2x - y = 3               and                x - 2y = 3

Solution:

To illustrate the problem, it is better to draw the graph of the given lines as follows:

Photo by Math Principles in Everyday Life

The two given lines form vertical angles, the acute angles and obtuse angles. Each angles are supplement to each other. There are two angle bisectors in these given lines, the angle bisector for the obtuse angle and the angle bisector for the acute angle. In this problem, we will find the equation of a line that bisects the acute angle. Let's assign a point that is located between the two given lines, and label this as P(x,y). From point P, draw a line that is perpendicular to 2x - y = 3 and label this as d₁. From point P, draw a line that is perpendicular to x - 2y = 3 and label this as d₂. 

Photo by Math Principles in Everyday Life

From the figure above, it shows a distance between a point to a line. The distance between a point to a line is given by the formula

Since we are finding the equation of the angle bisector, we have to equate the distance of a point to the two given lines, which are d₁ and d₂. Since point P is located below the line 2x - y = 3, then d₁ must be negative. Since point P is located above the line x - 2y = 3, then d₂ must be positive. 

Simplify the above equation

                           - 2x + y + 3 = x - 2y - 3

                             3x - 3y - 6 = 0

Divide both sides by 3

                             x - y - 2 = 0

Therefore, the equation of angle bisector, L is x - y - 2 = 0.

                             

Derivation - Tangent Law

Category: Trigonometry

"Published in Newark, California, USA"

On November 8, 2012, we derived the formulas for Sine Law and Cosine Law. Right now, we will derive the formula for Tangent Law.  Let's consider the formula for Sine Law:

In this derivation, we will use only the first two terms of the given equation above. Let's have this one

Arrange the above equation, we have

Add 1 on both sides of the equation and simplify

Subtract 1 on both sides of the equation and simplify

Divide equation 1 by equation 2

At the right side of the equation, use the Sum and Product of Two Angles Formula to convert the sum of the two angles into the product of two angles, we have

Therefore, 

If you will derive using the other term of Sine Law, the other formulas of Tangent Law will be