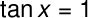Solving Trigonometric Equations, 6

Category: Trigonometry

"Published in Suisun City, California, USA"

Solve for the value of x for

Solution:

Consider the given equation above

Rewrite secant, tangent, and cotangent into its equivalent function as follows

Divide both sides of the equation by sin x, we have

but

and the above equation becomes

Take the 4th root at both sides of the equation

Consider the positive value

Take the inverse tangent at both sides of the equation

Consider the negative value

Take the inverse tangent at both sides of the equation

Therefore, the final answers are

   

where n is the number of revolutions.

Complex Fraction - Radicals

Category: Algebra

"Published in Suisun City, California, USA"

Simplify

Solution:

Consider the given equation above

Get the Least Common Denominator (LCD) of the numerator and the denominator of the given fraction and then simplify as follows

Get the reciprocal of the divisor and perform the multiplication, we have

Simplify the above equation and therefore, the final answer is

 

Algebraic Operations - Radicals, 27

Category: Algebra

"Published in Newark, California, USA"

Perform the indicated operations

Solution:

Consider the given equation above

The above equation can be written as

As a rule in Mathematics, all radicals in the denominator should be rationalized or eliminated. Eliminate the cube root sign at the denominator by applying the principles of Algebra which is the Sum and Difference of Two Cubes as follows

Apply the Distributive Property of Multiplication Over Addition at the numerator, we have

If you will multiply a radical with another radical with the same index, then the terms inside the radicals will be multiplied together.

How about if you will multiply a radical with another radical with different index? If their indexes are different, then you cannot multiply the terms inside the radicals together. The first two terms of the numerator at the above equation can be written as

At the first term of the numerator, the Least Common Denominator (LCD) of their fractional exponents is 12 (4 x 3). ¼ becomes 3/12 (12 ÷ 4 x 1 = 3) and ⅔ becomes 8/12 (12 ÷ 3 x 2 = 8).

At the second term of the numerator, the Least Common Denominator (LCD) of their fractional exponents is 12 (4 x 3). ¼ becomes 3/12 (12 ÷ 4 x 1 = 3) and ⅓ becomes 4/12 (12 ÷ 3 x 1 = 4).

Hence, the above equation becomes

Since the index of the two radicals at the first two terms of the numerator are now the same, then the terms inside the radicals can be multiplied together as follows

Therefore, the final answer is