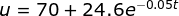"Published in Newark, California, USA"
The body of a murder victim was discovered at 11:00 pm. At 11:30 pm, the victim's body temperature was measured to be 94.6 °F. After 1 hour, the body temperature was 93.4 °F. The room where the body was found at a constant temperature of 70 °F. Assuming that Newton's Law of Cooling is applicable and assuming that the normal human body temperature is 37 °C, determine the time of death.
Solution:
This is a great application of Newton's Law of Cooling. Mostly the Federal Bureau of Investigation (FBI) and the police officers in United States of America are using this method to calculate the time of a death or murder. Even in other countries like Philippines, Canada, Mexico, Japan, Italy, China, and so on are using this method, too. Let's analyze the given word problem as follows
Let u = be the temperature of a dead body
t = be the time of death
k = constant of cooling/heating
According to Newton's Law, the time rate of change of temperature is proportional to the temperature difference.
The value of k is negative because it is a cooling process. When k is positive, then it is a heating process. The temperature of the surrounding is always a constant which is 70 °F. Solve for the general solution of the above equation, we have
Integrate on both sides of the equation
Take the inverse natural logarithm on both sides of the equation
but eC is still a constant. The above equation becomes
To solve for the values of k and C, we need to get the values of the limits as follows
If u1 = 94.6 °F, then t1 = 0 (measured at 11:30 pm)
u2 = 93.4 °F, then t2 = 1.0 hr (measured at 12:30 am)
Substitute the first limit to the above equation to solve for the value of C
Therefore,
Take natural logarithm on both sides of the equation
Next, we need to use the normal temperature of a body (u = 37 °C = 98.6 °F) in order to calculate the time of death of a person as follows
Since the value of time is negative, then we have to subtract it from the time where a dead body was first measured, which is at 11:30 pm, as follows
11:30 ------> 11:30:00 -------> 11:29:60
- 3:00:48 - 3:00:48 - 3:00:48
---------------
8:29:12
Therefore, a person is dead at 8:29:12 pm.